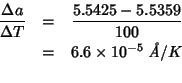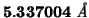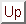


Next: 19981126: same as 19981124,
Up: MD work on
Previous: 19981119: going on with
19981124:
 , rigid ions
, rigid ions
Simulation of the system:
 .
.
I've run GULP with the following occupancies, corresponding to the
composition of the system being simulated:
############
# Cores
############
Ce4 core 0.000000 0.000000 0.000000 -3.700000 0.35185185
Ce3 core 0.000000 0.000000 0.000000 -4.700000 0.14814815
Zr core 0.000000 0.000000 0.000000 2.65 0.50
O core 0.250000 0.250000 0.250000 0.07700 0.96296296
############
# Shells
############
Ce4 shel 0.000000 0.000000 0.000000 7.700000 0.35185185
Ce3 shel 0.000000 0.000000 0.000000 7.700000 0.14814815
Zr shel 0.000000 0.000000 0.000000 1.35 0.50
O shel 0.250000 0.250000 0.250000 -2.07700 0.96296296
From the structure optimization, I've got the value of
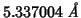 for the lattice constant.
for the lattice constant.
Then, I've applied a correction for thermal expansion, based on the
data in the paper [3]. From table 2 of the paper one
gets a value of
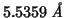 for the lattice constant of
defective
for the lattice constant of
defective 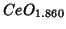 at
at 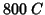 . From table 3 the value of
. From table 3 the value of
 is taken for defective
is taken for defective 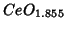 at
at
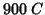 . Hence, the following thermal expansion coefficient can be
assumed:
. Hence, the following thermal expansion coefficient can be
assumed:
Approximations:
- The paper [3] is about defective and
stoichiometric pure ceria, while my system is a solid
solution with zirconia
- Even by considering
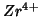 and
and 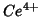 as being the
same, the formula for my defective system turns out to be
as being the
same, the formula for my defective system turns out to be
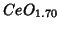 , which is different from
, which is different from 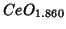 or
or
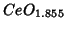 in the paper: these latter are the compositions nearest to
mine which can be found in tables 2 and 3
in the paper: these latter are the compositions nearest to
mine which can be found in tables 2 and 3
- I assume a constant thermal expansion coefficient
Applying the correction for thermal expansion gives:
( is the temperature of the simulation)
is the temperature of the simulation)
This was the value used in the input to the genlat program.
Nov 30 (Mon), 1998
In the light of the results obtained in 19981126, which look much better
than those in this calc and which were obtained by simply reducing the
lattice constant of the fluoritic structure, I was thinking that the
GULP minimization had to be performed with rigid cations, like the
MD calc. So I've run GULP with rigid cations to see if the
optimized lattice constant was different: alas! no success. I got
exactly the same value as the one with polarizable cations:
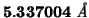
The program was killed prematurely because of a time limit overflow. Anyhow, it
almost completed its task, writing successfully 4420 configurations (I
set out things in the CONTROL file in order to write 5000
configurations). So we can safely consider the calculation as
completed.
Sadly, the MSD plot is not good.



Next: 19981126: same as 19981124,
Up: MD work on
Previous: 19981119: going on with
![]() for the lattice constant.
for the lattice constant.
![]() for the lattice constant of
defective
for the lattice constant of
defective ![]() at
at ![]() . From table 3 the value of
. From table 3 the value of
![]() is taken for defective
is taken for defective ![]() at
at
![]() . Hence, the following thermal expansion coefficient can be
assumed:
. Hence, the following thermal expansion coefficient can be
assumed: